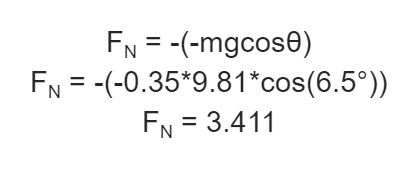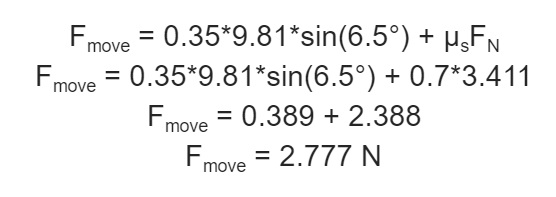Goliath Fall 2016
Torque Calculation for Going Up a Ramp
By: Diana Nguyen (Missions, Systems, and Test Engineer)
Approved by Kristen Oduca (Project Manager)
Introuction
Requirement: To drive the Goliath up the 6 degree incline, the motor shall provide a minimum torque of 0.0249 Nm.
For the Save the Human Game, there will be two ramps in the course of the game. Both ramps will have a 6.5° incline, plateaus and then decline 6.5°. Goliath will need to be able follow the biped up the ramp and across it.
Calculations
Goliath needs to be able to go up a ramp with an incline of 6.5°. The mass of Goliath is 350 g or 0.35 kg and the static coefficient of friction for rubber and cardboard is between 0.5-0.8 [1]. We need to be able to produce enough force to be able to move the Goliath up the ramp which would be Fmove.
Figure 1 – Fmove Equation
From Figure 1 we can see that FN is the opposite force of -mgcosθ. Therefore we can assume FN = -(-mgcosθ).
Figure 2 – FN Equation
Since we now have FN and we know µs ,which is the the static friction. We can complete our calculation of Fmove.
Figure 3 – Calculating Fmove
Using this we can now calculate the amount of torque the motors need to produce. Torque can be calculated from Torque = (Radius of shaft) * Fmove.
Figure 5 shows the diagram of the forces.
Figure 5 – Diagram of Forces on a Ramp
Conclusion
Base off these calculations we can conclude that based off our current model Goliath we need a motor with a minimum torque of 0.0528 Nm. After our motor test we can see if the motors we purchased be able to produce this minimum amount required to go up the ramp.